
These are helpful for any problem that uses the phrases "as many as". You could also use this to learn to solve those one-step problems.Ĭ) Ratio Models -This is used to compare multiplication or division scenarios. We could recreate this situation by using either: This is a great way to begin to use algebra to model situation because this model allows us to approach these equations from both angles. This allows us to setup a comparison using either addition or subtraction. For example, let’s compare the number of waters to the number of sodas, from the previous scenario that we described. That section represents the value we are attempting to determine. In most cases we are using two tapes with one section missing. With this tape diagram we are comparing two different quantities by using those math operations. We could use that information to model: x + 4 + 2 = 12.ī) Addition Comparison - The model is used to help in situations of addition or subtraction. If we were not told the number of waters but knew there were 12 drinks in the cooler. For example, building off of what we have explored so far. This model can also be used to make visuals of basic algebraic equation. We can use this to learn that there are a total of 12 drinks in the cooler. The rectangle for 6 is bigger than the rectangle for 4 or 2. Here is a look at a tape diagram that models the types of drinks in your cooler if the values were 6 waters, 4 juices, 2 sodas:Īs you can see from the diagram the length of the rectangle is in sync will the size of the value. These can be used to model all of the four basic math operations (addition, subtraction, multiplication, and division). It almost acts like a pie or circle chart in a rectangular format.

There are commonly three main uses of tape diagrams:Ī) Part Whole Model - This is a quick way to display multiple items that contribute towards a whole. This serves as a great visual reminder of all the aspects found in a word problem. Each rectangle usually represents a section of a ratio. They use various rectangular arrays in drawing formats to recreate these situations. Tape diagrams are mathematical graphic organizers that students can create to model many different types of real-world math situations. You will work on problems like this: Lisa raised $45 for the animal shelter, which was 5 times as much Practice Sheet 5 - These questions are more focused on reading premade diagrams.Practice Sheet 4 - Students vote for what they want to eat during a class party.Practice Sheet 3 - Proportions on the length of ribbons and wooden boards.How much blue paint would they need to make 16 gallons of purple paint? Homework 2 - Painters use a ratio of 5 parts blue paint to 3 parts red paint when mixing the purple paint for Vikings Stadium.Homework 1 - This sheet is the best mix of problems in the entire set.How many students would be on each school Example: It took 4 buses to take 120 students on a field trip. Quiz - We fire 6 problems at you from all directions.Answer Keys - These are for all the unlocked materials above.Īnswers are included in these pages.Making and Reading Diagrams Practice 2- Remember these are used vehicles.Making Tape Diagrams Practice Worksheet - All these problems ask kids to make their own diagrams.Guided Lesson Explanation - We test both skills here.Guided Lesson - How much money did Peter go to the store with? How many runs did Rich account for?.
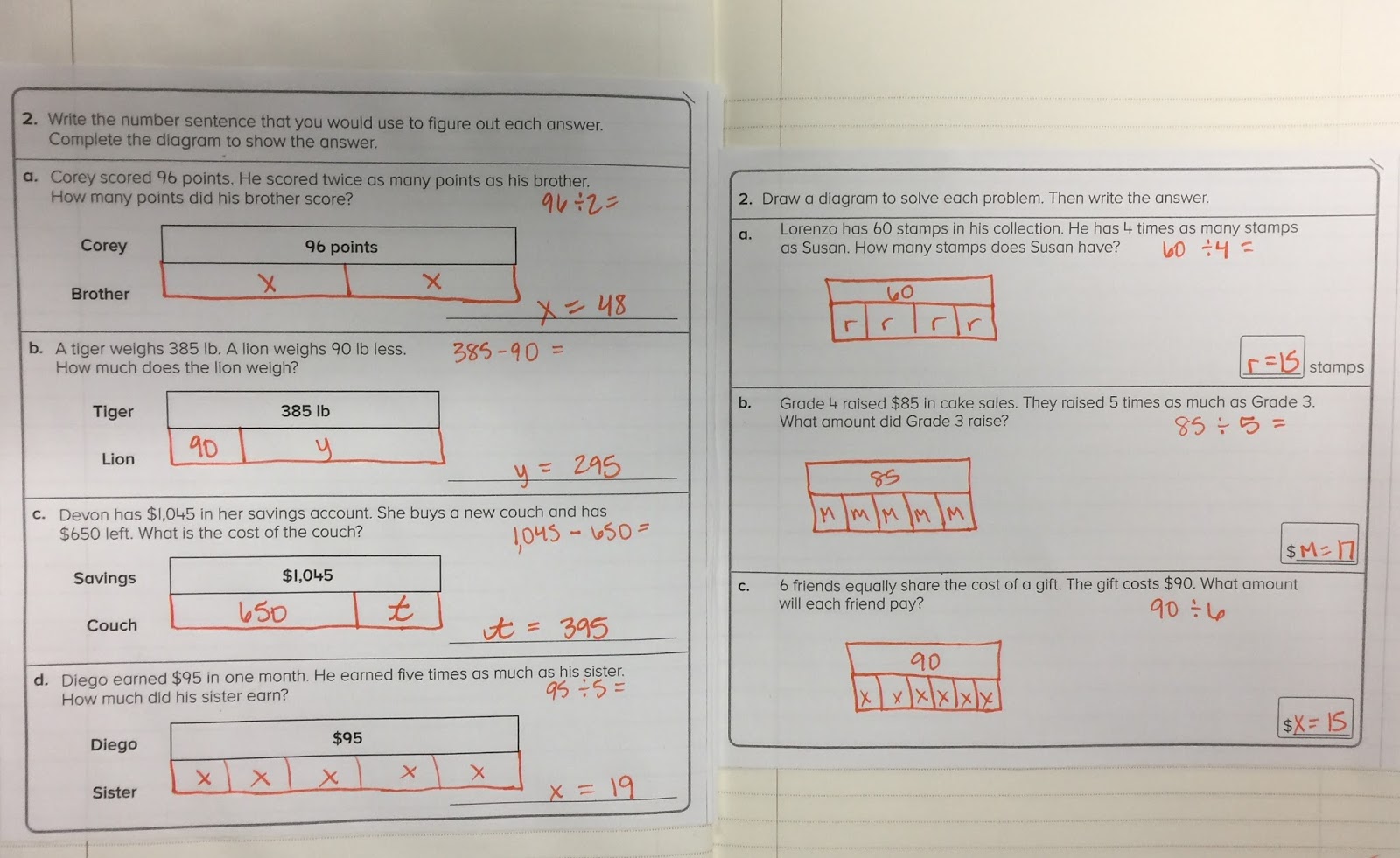
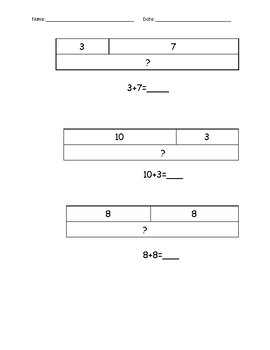
The basic premise is that we are using visual diagrams (pieces of tape or bars) to define parts of a whole. You may also see this work referred to as a Bar Model.


 0 kommentar(er)
0 kommentar(er)
